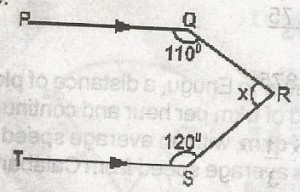
1991 - JAMB Mathematics Past Questions and Answers - page 5
From a point Z, 60 m north of X, a man walks 60√3m eastwards to another point Y. Find the bearing of Y from X.
Find the total area of the surface of a solid cylinder whose base radius is 4cm and height is 5cm
3% of a family's income is spent on electricity, 58% on food, 20% on transport, 11% on education and 7% on extended family. The angles subtended at the centre of the pie chart under education and food are respectively
Fifty boxes each of 50 bolts were inspected for the number which were defective. The following was the result
(\begin{array}{c|c} \text{No. defective per box} & 4 & 5 & 6 & 7 & 8 & 9 \ \hline \text{No. of boxes} & 2 & 7 & 17 & 10 & 8 & 6\end{array})
The mean and the median of the distribution are respectively
Fifty boxes each of 50 bolts were inspected for the number which were defective. The following was the result
(\begin{array}{c|c} \text{No. defective per box} & 4 & 5 & 6 & 7 & 8 & 9 \ \hline \text{No. of boxes} & 2 & 7 & 17 & 10 & 8 & 6\end{array})
Find the percentage of boxes containing at least 5 defective bolts each
A crate of soft drinks contains 10 bottles of Coca-cola, 8 of Fanta and 6 of sprite. If one bottle is selected at random, what is the probability that it is NOT a Cocacola bottle?