2004 - JAMB Mathematics Past Questions and Answers - page 1

\( ARC\hspace{1mm}length = (\frac{\theta}{360})\times 2\pi r\22=\frac{3x}{360}\times \left(2 \times(\frac{22}{7})\times(\frac{7}{1})\right)\3x = 180\x = \frac{180}{3}\x = 60^{\circ}\)

\(PQ = \sqrt{(β - 1)^{2} + (1 - α)^{2}}\\ 3 =\sqrt{(β^{2} -2β^{2} + 1 + 1 - 2α + α^{2})}\\ 3 = \sqrt{(α^{2} + β^{2} - 2α + 2β + 2)}\\ 3 = \sqrt{(α^{2} + β^{2} - 2(α + β) + 2)}\\ 3 = \sqrt{(α^{2} + β^{2} - 2 * 2 + 2)}\\ 3 = \sqrt{(α^{2} + β^{2} - 2)}\\ 9 = (α^{2} + β^{2} - 2)\\ α^{2} + β^{2} = 9 + 2\\ α^{2} + β^{2} = 11\)

6x + 6y = (n - 2) 180
6x + 6y= (5 - 2) 180
6(x + y) = 3 * 180
x + y = (3 * 180)/6
x + y = 90^o
y = 90 - x

Area of parallelogram PQTU = base * height
32 = 4 * h
w = 32/4
w = 8
∴ = Area of Trapezium PQRU = (1/2)(4 + 14) *8
= 1/2 * (18 * 8)
= 72 cm²

\(Mid point = \frac{(x_1 + x_2)}{2} ; \frac{(y_1 + y_2)}{2}\\ = \frac{(-3 + 5)}{2} ; \frac{(5 - 3)}{2}\\ = \frac{2}{2} ; \frac{2}{2}\\ = (1, 1)\)

The diagonal QS bisects the angle formed by PQ and QR
∴ [A]

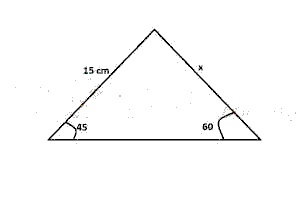
\(\frac{X}{sin45}=\frac{15}{sin60}\X=\frac{15sin45}{sin60}\X=\frac{15\times(\frac{1}{\sqrt{2}})}{\sqrt{\frac{3}{2}}}\X=\frac{15}{\sqrt{2}}\times\frac{2}{\sqrt{3}}\X=\frac{30}{\sqrt{6}}\X=\frac{30}{\sqrt{6}}\times\frac{\sqrt{6}}{\sqrt{6}}=\frac{30\sqrt{6}}{6}=5\sqrt{6}\)

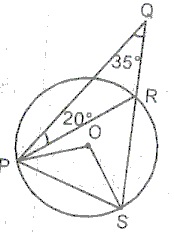
x^o = 35 + 29 (Exterior angle = sum of two interior opposite angles)
x = 55^o (∠ at the center twice ∠ at circumference) y = 110^o
∠PSO = ∠SPO (base ∠S of 1sc Δ b/c PO = SO)
∴ ∠PSO = (180 - 110)/2
= 35^o

The locus of a point which is 5cn from the line LM is a pair of lines on opposite sides of LM and parallel to it, each distance 5cm from LM

y = 3cos(x/3)
dy/dx = 3x(1/3)x - sin (x/3)
= - sin x/3
But x = 3π/2 ∴ -sin(x/3) = -sin(3π/6)
= -sin (3 * 180)/6
= - sin 90
= -1