2007 - JAMB Mathematics Past Questions and Answers - page 4
31
The sum of the ages of Musa and Lawal is 28 years. After sharing a certain sum of money in the ratio of their ages, Musa gets N600 and Lawal N800. How old is Lawal?
A
14 years
B
20 years
C
12 years
D
16 years
correct option: d
M + L = 28,
M : L = 600 : 800
= 3 : 4
\(\frac{M}{L}\) = \(\frac{3}{4}\) \(\to\) M = \(\frac{3}{4}\)L
\(\frac{3}{4}\)L + L = 28
\(\frac{7L}{4}\) = 28
L = \(\frac{4 \times 28}{7}\)
= 16
Users' Answers & CommentsM : L = 600 : 800
= 3 : 4
\(\frac{M}{L}\) = \(\frac{3}{4}\) \(\to\) M = \(\frac{3}{4}\)L
\(\frac{3}{4}\)L + L = 28
\(\frac{7L}{4}\) = 28
L = \(\frac{4 \times 28}{7}\)
= 16
32
x10 = 12145 find x.
A
124
B
184
C
121
D
180
correct option: b
x10 = 1214 5, 1 x 53 + 2 x 52 + 1 x 51 + 4 x 5o
= 125 + 50 + 5 + 4 18410
x = 184
Users' Answers & Comments= 125 + 50 + 5 + 4 18410
x = 184
33
If log102 = x, express log10 12.5 in terms of x
A
2(1 - x)
B
2(1 + x)
C
2 - 3x
D
2 + 3x
correct option: c
log10 12.5 = log10\(\frac{25}{2}\)
= log10\(\frac{100}{8}\) = log10 100 - log108
= log10 102 - log102
= 2log10 10 - 3log102
= 2 - 3x
Users' Answers & Comments= log10\(\frac{100}{8}\) = log10 100 - log108
= log10 102 - log102
= 2log10 10 - 3log102
= 2 - 3x
34
A
\(\frac{5}{30}\)
B
\(\frac{11}{30}\)
C
\(\frac{17}{30}\)
D
\(\frac{1}{30}\)
correct option: c
Total fruits 6 + 11 + 13 = 30,
prob. (Grape) = \(\frac{6}{30}\)
prob. (Banana) = \(\frac{11}{30}\)
= \(\frac{11}{30}\) + \(\frac{6}{30}\) = \(\frac{17}{30}\)
Users' Answers & Commentsprob. (Grape) = \(\frac{6}{30}\)
prob. (Banana) = \(\frac{11}{30}\)
= \(\frac{11}{30}\) + \(\frac{6}{30}\) = \(\frac{17}{30}\)
35
A
69
B
29
C
78
D
38
correct option: b
No. of students that made the trip
= 2 + 3 = 5 = 6 = 8 = 4 + 1 = 29
Users' Answers & Comments= 2 + 3 = 5 = 6 = 8 = 4 + 1 = 29
36
A
y = x3 - 4x2 + 5x - 2
B
y = x3 - 3x - 2
C
y = x3 - 4x + 2
D
y = x3 + 2x2 - x - 2
correct option: d
The roots of the graph are -2, -1 and 1
y = (x + 2)(x + 1)(x - 1) = (x + 2)(x2 - 1)
= x3 + 2x2 - x - 2
Users' Answers & Commentsy = (x + 2)(x + 1)(x - 1) = (x + 2)(x2 - 1)
= x3 + 2x2 - x - 2
37
If 5, 8, 6 and 2 occur with frequencies 3, 2, 4 and 1 respectively. Find the product of the modal and the median number
A
36
B
48
C
30
D
40
correct option: a
Arrange in ascending order
2, 5, 5, 6, 6, ,6, 6, 8, 8
Position of median = N/2 = 8/2 = 4th and 5th
∴Median = (6+6) / 2 = 12/2 = 6
Mode the item that repeat itself most = 6
∴Product of modal and median number = 6 x 6 = 36
Users' Answers & Comments2, 5, 5, 6, 6, ,6, 6, 8, 8
Position of median = N/2 = 8/2 = 4th and 5th
∴Median = (6+6) / 2 = 12/2 = 6
Mode the item that repeat itself most = 6
∴Product of modal and median number = 6 x 6 = 36
38
In a basket, there are 6 grapes, 11 bananas and 13 oranges. If one fruit is chosen at random. What is the probability that the fruit is either a grape or a banana
A
6/30
B
5/30
C
17/30
D
11/30
correct option: c
Number of grapes = 6
Number of banana = 11
Number of oranges = 13
Total = 30
P(Grape) = 6/30, P(Banana) = 11/30
P(Orange) = 13/30
∴P(Either grape or banana) = 6/30 + 11/30
= 17/30
Users' Answers & CommentsNumber of banana = 11
Number of oranges = 13
Total = 30
P(Grape) = 6/30, P(Banana) = 11/30
P(Orange) = 13/30
∴P(Either grape or banana) = 6/30 + 11/30
= 17/30
39
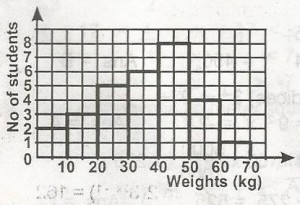
The histogram above represents the weights of students who travelled out to their school for an examination. How many people made the trip.
A
78
B
38
C
29
D
69
correct option: c
Users' Answers & Comments40
A senatorial candidate had planned to visit seven cities prior to a primary election. However, he could only visit four of the cities. How many different itineraries could be considered?
A
640
B
840
C
520
D
920
correct option: b
Number of itineraries = 7P4
=\(\frac{7!}{(7-4)!}\\ =\frac{7!}{3!}\\ =\frac{7 \times 6 \times 5 \times 4 \times 3!}{3!}\\ =840\)
Users' Answers & Comments=\(\frac{7!}{(7-4)!}\\ =\frac{7!}{3!}\\ =\frac{7 \times 6 \times 5 \times 4 \times 3!}{3!}\\ =840\)