2016 - JAMB Mathematics Past Questions and Answers - page 1
1
Integrate \(\frac{2x^3 + 2x}{x}\) with respect to x
A
\(\frac{2x^3}{3}\) - 2x + k
B
x3 + 2x + k
C
\(\frac{2x^3}{3}\) + 2x + k
D
x3 - 2x + k
correct option: c
\(\int\) \(\frac{2x^3 + 2x}{x}\) = \(\int\) \(\frac{2x^3}{x}\) + \(\frac{2x}{x}\) = \(\int\) (2x2 + 2) dx
\(\frac{2x^2+1}{2 + 1}\) + 2x + k
= \(\frac{2x^3}{3}\)+ 2x + k
Users' Answers & Comments\(\frac{2x^2+1}{2 + 1}\) + 2x + k
= \(\frac{2x^3}{3}\)+ 2x + k
2
If the mean of 4, y, 8 and 10 is 7. Find Y?
A
6
B
10
C
7
D
9
correct option: a
\(\frac{4 + y + 8 + 10}{4}\) = 7
22 + y = 4 x 7
22 + y = 28
y = 28 - 22 = 6
Users' Answers & Comments22 + y = 4 x 7
22 + y = 28
y = 28 - 22 = 6
3
Find the derivative of y = ( \(\frac{1}{3}\)X + 6)
A
2( \(\frac{1}{3}\)X + 6
B
\(\frac{2}{3}\) ( \(\frac{1}{3}\)X + 6)
C
\(\frac{2}{3}\) ( \(\frac{1}{3}\)X + 6)2
D
\(\frac{1}{3}\) ( \(\frac{1}{3}\)X + 6)2
correct option: b
Y = ( \(\frac{1}{3}\)X + 6)2
\(\frac{dy}{dx}\) = 2( \(\frac{1}{3}\)X + 6) \(\frac{1}{3}\)
= \(\frac{2}{3}\) ( \(\frac{1}{3}\)X + 6)
Users' Answers & Comments\(\frac{dy}{dx}\) = 2( \(\frac{1}{3}\)X + 6) \(\frac{1}{3}\)
= \(\frac{2}{3}\) ( \(\frac{1}{3}\)X + 6)
4
In a school of 150 students, 80 offer French while 60 offer Arabic and 20 offer neither. How many students offer both subjects?
A
45
B
10
C
35
D
30
correct option: b
80 - x + x + 60 - x + 20 = 150
160 - x = 150
x = 160 - 150 = 10
Users' Answers & Comments160 - x = 150
x = 160 - 150 = 10
5
If the 2nd term of a G.P is \(\frac{8}{9}\) and the 6th term is 4\(\frac{1}{2}\). Find the common ratio.
A
2
B
\(\frac{3}{2}\)
C
\(\frac{2}{3}\)
D
3
correct option: b
T2 = \(\frac{8}{9}\), T6 = 4\(\frac{1}{2}\)
ar = \(\frac{8}{9}\)
ar5 = \(\frac{9}{2}\)
\(\frac{ar}{ar^5}\) = \(\frac{8}{9}\) x \(\frac{2}{9}\)
\(\frac{1}{r^4}\) = \(\frac{16}{81}\)
r = \(\sqrt[4]{18/16}\) = \(\frac{3}{2}\)
Users' Answers & Commentsar = \(\frac{8}{9}\)
ar5 = \(\frac{9}{2}\)
\(\frac{ar}{ar^5}\) = \(\frac{8}{9}\) x \(\frac{2}{9}\)
\(\frac{1}{r^4}\) = \(\frac{16}{81}\)
r = \(\sqrt[4]{18/16}\) = \(\frac{3}{2}\)
6
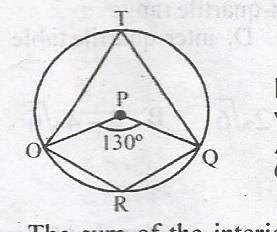
A
230o
B
55o
C
115o
D
65o
7
A
5
B
7
C
6
D
8
correct option: d
\(\frac{(n - 2) 180}{180}\) = \(\frac{1080}{180}\)
(n - 2) = 6
n = 6 + 2 = 8
Users' Answers & Comments(n - 2) = 6
n = 6 + 2 = 8
8
In the diagram above, l1 is parallel to l2, Find the value of < PMT
A
82o
B
36o
C
72o
D
118o
correct option: a
< MPT = 180o - 118o = 62o
< PML = 62o ( Alternative angle)
y + 2y + 10o + 62o = 180o (Angles on a straight line)
3y = 180 - 72
\(\frac{3y}{3}\) = \(\frac{108}{3}\)
y = 36o
< PMT = 2y + 10 = 2(36) + 10 = 82o
Users' Answers & Comments< PML = 62o ( Alternative angle)
y + 2y + 10o + 62o = 180o (Angles on a straight line)
3y = 180 - 72
\(\frac{3y}{3}\) = \(\frac{108}{3}\)
y = 36o
< PMT = 2y + 10 = 2(36) + 10 = 82o
9
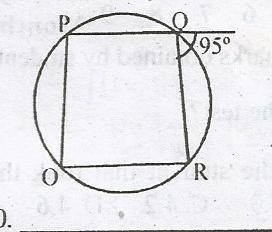
A
110o
B
70o
C
95o
D
85o
10
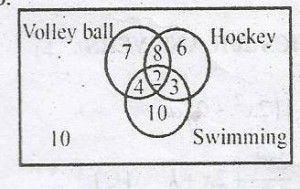
A
15
B
16
C
20
D
18
correct option: a
Number of students that plays only two games = 4 + 8 + 3 + = 15
Users' Answers & Comments