2016 - JAMB Mathematics Past Questions and Answers - page 5
41
If Q is a factor of 18 and T is prime numbers between 2 and 18. What is Q\(\cap\)T?
A
(2,3)
B
(2,3,18)
C
(2,3,9)
D
(2,3,6)
correct option: a
Q = {1,2,3,6,9,18}, T = {2,3,5,7,11,13,17}
Q\(\cap\)T = {2,3}
Users' Answers & CommentsQ\(\cap\)T = {2,3}
42
A
60o
B
80o
C
70o
D
50o
43
Find the mean of 10, 8, 5, 11, 12, 9, 6, 3, 15, and 23.
A
11.2
B
10
C
10.2
D
11.3
correct option: c
\(\frac{10 + 8 + 5 + 11 + 12 + 9 + 6 + 3 + 15 + 23}{10}\)
= \(\frac{102}{10}\) = 10.2
Users' Answers & Comments= \(\frac{102}{10}\) = 10.2
44
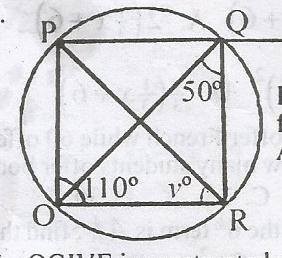
A
70o
B
20o
C
50o
D
30o
45
OGIVE is constructed using
A
Third quartile range
B
Semi-quartile range
C
Cummulative frequency table
D
Inter-quartile table
correct option: c
Users' Answers & Comments46
Rationalize \(\frac{\sqrt{6} - \sqrt{4}}{\sqrt{6} + \sqrt{4}}\)
A
5 + 2 \(\sqrt{6}\)
B
5 - 4 \(\sqrt{6}\)
C
5 + 4 \(\sqrt{6}\)
D
5 - 2 \(\sqrt{6}\)
correct option: d
\(\frac{\sqrt{6} - \sqrt{4}}{\sqrt{6} + \sqrt{4}}\) = \(\frac{6-2\sqrt{6} - 2\sqrt{6} + 4}{6 - 4}\)
\(\frac{10 - 4\sqrt{6}}{2}\) = 5 - 2\(\sqrt{6}\)
Users' Answers & Comments\(\frac{10 - 4\sqrt{6}}{2}\) = 5 - 2\(\sqrt{6}\)
47
How many students in all took the test?
A
40
B
30
C
10
D
20
48
A
4.5
B
4.3
C
4.2
D
4.6
correct option: c
(3 x 0) + (1 x 2) + (5 x 2) + (5 x 3) + (5 x 4) +
(10 x 5) + (4 x 6) + (3 x 7) + (1 x 8) + (2 x 9) \(\div\) 40
= \(\frac{168}{40}\) = 4.2
Users' Answers & Comments(10 x 5) + (4 x 6) + (3 x 7) + (1 x 8) + (2 x 9) \(\div\) 40
= \(\frac{168}{40}\) = 4.2
49
A
\(\frac{1}{3}\)
B
\(\frac{1}{5}\)
C
\(\frac{1}{4}\)
D
\(\frac{1}{6}\)
correct option: d
\(\theta\) = 180o -(90 + 60)
\(\theta\) = 180o - 150o = 30o
Fraction of shaded position = \(\frac{30}{360}\) + \(\frac{30}{360}\)
= \(\frac{1}{12}\) + \(\frac{1}{12}\) = \(\frac{1}{6}\)
Users' Answers & Comments\(\theta\) = 180o - 150o = 30o
Fraction of shaded position = \(\frac{30}{360}\) + \(\frac{30}{360}\)
= \(\frac{1}{12}\) + \(\frac{1}{12}\) = \(\frac{1}{6}\)
50
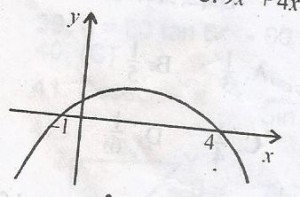
A
x\(\leq\)-1, x \(\geq\)4
B
x\(\leq\)4
C
-1\(\leq\)x\(\leq\)4
D
x\(\leq\)-1, o\(\leq\)x\(\leq\)4