1994 - JAMB Mathematics Past Questions and Answers - page 5

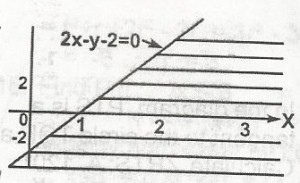
2x - y - 2 \(\geq\) 0 = y \(\leq\) 2x - 2

when x = 0, y = -2, when y = 0, x = 1

SQR + RQV + VQU = 18^o angle on a straight line SP is parallel to QR and PV is parallel to TR

< STP = < RQV = 30^o

But SQR + 30^o + 50^o = 180^o

SQR = 180 - 80

= 100^o

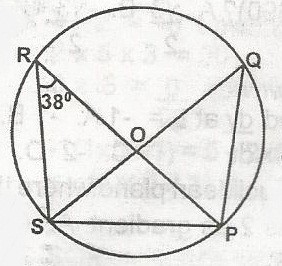
< SRP = < SQP = 38^o (angle in the same segment of a circle are equal)

But < SPQ = 90^o (angle in a semicircle)

also < PSQ + < SQP + < SPQ = 180^o (angles in a triangle = 180^o)

< PSQ + 38^o + 90^o = 190^o

< PSQ = 128^o = 180^o

PSQ = 180^o - 128^o

PSQ = 52^o

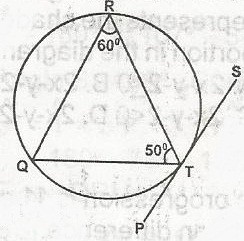
RTS = RQT (angle between a tangent and a chord at the point of contact is equal to the angle in the alternate segment) But R = Q + T = 180

RQT = 180^o - (50 + 60)

= 180^o - 110^o

= 70^o

Since RQT = RTS = 70^o

A_{\(\bigtriangleup\)} = \(\sqrt{S(S - a) (S - b)(S - c)}\) (Hero's Formula)

S = \(\frac{a + b + c}{2}\) = \(\frac{5 + 6 + 7}{2}\)

\(\frac{18}{2} = 9\)

A_{\(\bigtriangleup\)} \(\sqrt{9} \times 4 \times 3 \times 2\)

\(\sqrt{216} = 6 \sqrt{6}cm^3\)

A_{\(\bigtriangleup\)} = \(\frac{1}{2} \times 6 \times h\)

6\(\sqrt{6} = \frac{1}{2} \times 7 \times h\)

h = \(\frac{12}{h} \sqrt{6}\)

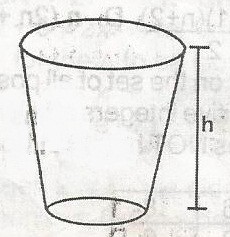
\(\frac{x}{r}\) = \(\frac{x + h}{2r}\)

2 x r = r (x + h)

Total height of cone = x + h

but x = h

total height = 2h

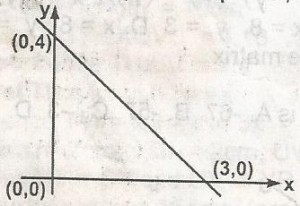
Gradient of line = \(\frac{\text{Change in y}}{\text{Change in x}} = \frac{y_2 - y_1}{x_2 - x_1}\)

y₂ = 0, y₁ = 4

x₂ = 3 and x₁ = 0

\(\frac{y_2 - y_1}{x_2 - x_1} = \frac{0 - 4}{3 - 0} = \frac{-4}{3}\)

Equation of straight line = y = mx + c

where m = gradient and c = y

intercept = 4

y = 4x + \(\frac{4}{3}\), multiple through by 3

3y = 4x + 12