2008 - JAMB Mathematics Past Questions and Answers - page 5

X = ²⁰/₄
= 5
mean deviation = ⁸/₄ = 2

ACCEPTANCE = 10 Letters
A = 2 letters
C = 3 letters
E = 2 letters
Can be arranged in 10! / (2!3!2!) ways

\(^{10}C_6 = \frac{10!}{(10-6)!6!}\\ =\frac{10!}{4!6!}\\ =\frac{10\times 9\times 8 \times 7 \times 6!}{4\times 3\times 2\times 1 \times 6!}\\ =210\)

OBSTRUCTION
Total possible outcome = 11
Number of chance of getting T = 2
P(picking T) = ²/₁₁

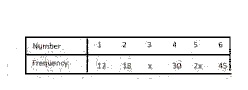
Total possible outcome
12+18+x+30+2x+45 = 105+3x
∴105+3x = 150
3x = 150-105
3x = 45
x = 15
P(obtaining 5) = \(\frac{2x}{(105+3x)}But x= 15\\ =\frac{2(15)}{(105+3(15))}\\ =\frac{30}{(105+45)}\\ =\frac{30}{150}\\ =\frac{1}{5}\)

If 125_x = 20₁₀, find x

125_x = 1 x 3² + 2 x 3¹ + 5 x 3⁰

= 1 x 9 + 2 x 3 + 5 x 1

= 9 + 6 + 5

= 20

therefore, 125₃ = 20₁₀

x = 3

\(\frac{\frac{3}{8} \div \frac {1}{2} - \frac{1}{3}}{\frac{1}{8} \times \frac {2}{3} + \frac{1}{3}}\)

= \(\frac{\frac{3}{8} \times \frac {2}{1} - \frac{1}{3}}{\frac{1}{8} \times \frac {2}{3} + \frac{1}{3}}\)

= \(\frac{\frac{6}{8} - \frac{1}{3}}{\frac{2}{24} + \frac{1}{3}}\)

= \(\frac{\frac {18 - 8}{24}}{\frac{2 + 8}{24}}\) = \(\frac{\frac{10}{24}}{\frac{10}{24}}\)

= \(\frac{10}{24}\) \(\div\) \(\frac{10}{24}\)

= \(\frac{10}{24}\) x \(\frac{24}{10}\) = 1

Express 123456 to 3 significant figures

= 123 000

Calculate the simple interest on N7500 for 8 years at 5% per annum

S.I = \(\frac{P \times R \times T}{100}\)

S.I = \(\frac{7500 \times 5 \times 8}{100}\)

S.I = \(\frac{300000}{100}\)

S.I = N3000