2009 - JAMB Mathematics Past Questions and Answers - page 3

m⊗n = m + n + mn

for inverse -5^-1 x -5 = e

-5^-1 + -5 + (-5^-1)(-5) = 0

-5 + (-5^-1(-5)) = 5

-5^-1.(1 + -5) = 5

-5^-1(-4) = 5

-5^-1 = -⁵/₄

Q = ( \left[ \begin{array}{ccc}

9 & -2 \

-7 & 4 \

\end{array} \right])

|Q| = 9x4 - (-2x7)

= 36 - 14

= 22

(P=\left[\begin{array}{cc}x+3 & x+2\

x+1 & x-1\end{array}\right]) evaluate x if |P| = -10

(x+3)(x-1) - {(x+1)(x+2)} = -10

x² - x + 3x - 3 - {x² + 2x + x + 2} = -10

x² + 2x - 3 - {x² + 3x + 2} = -10

-x - 5 = -10

-5 + 10 = x

5 = x

∴x = 5

y = x, Gradient (m₁) = 1

y = √3x gradient (m₂) = √3

(tan\theta = \frac{m_2 - m_1}{1+m_2 m_1}\

tan\theta = \frac{\sqrt{3}-1}{1+\sqrt{3}\times 1}\

=\frac{1.73 -1 }{1+1.73}=

\frac{0.73}{2.73}=0.268)

θ = Tan^-1 (0.268)

= 15^o

Sum of interior angles of a polygon = each interior ∠ x n

(n-2)180 = 150 x n

180n - 360 = 150n

180n-150n = 360

30n = 360

n = 360/30

n = 12 sides

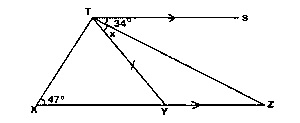
In Δ TYX

XY = TY ∴y =47^o base ∠s of ISCΔ

But y+x+34+47 = 180 interior

opposite ∠s are supplementary

47 + x + 34 + 47 = 180

x + 128= 180

x = 180- 128

x = 52^o

X² + x² = 2²

2x² = 4

x² = ⁴/₂

x² = 2

x = +/-√2

Area of Δ = ¹/₂bh

= ¹/₂ x √2 x √2

= ²/₂

= 1 cm²

x² + 5² = 13²

x² + 25 = 169

x² = 144

x = √ 144 

= 12

Length of the chord AB = 2x

2*12= 24cm

Locus of a particle which moves in the first quadrant so that it is equidistant from the lines

X = 0 and Y = 0 and x = 0 and y = 0 are the x and y axes is the lines bisecting the angle between x and y axes