1992 - JAMB Mathematics Past Questions and Answers - page 3

Q = (\begin{vmatrix} x & 2 & z \ 3 & y & 2\ 0 & 3 & z \end{vmatrix}) Where p^T is the transpose P calculate /p^T/ when x = 0, y = 1 and z = 2

p = (\begin{vmatrix} x & 3 & 0 \ 2 & y & 3\ 4 & 2 & 4 \end{vmatrix})

Q = (\begin{vmatrix} x & 2 & z \ 3 & y & 2\ 0 & 3 & z \end{vmatrix})

PQ is equivalent to

If the angles of quadrilateral are (P + 10)^o(2P - 30)^o(3P + 20)^o and 4p^o, find p

Determine the distance on the earth's surface between two town P (lat^oN, Long 20^oN) and Q(Lat 60^oN, Long 25^oW) (Radius of the earth = 6400km)

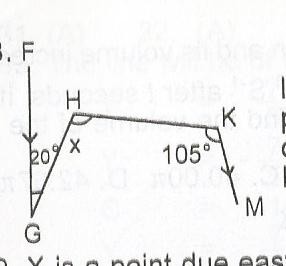
If in the diagram, FG is parallel to KM, find the value of x

x is a point due east of point Y on a coast Z is another point on the coast but 63m due south of y. If the distance ZX is 12Km. Calculate the bearing of Z from X

The locus of a point which is equidistant from two given fixed points is the

What is the perpendicular distance of a point (2, 3) from the line 2x - 4y + 3 = 0?

find then equation line through (5, 7) parallel to the line 7x + 5y = 12

Given that (\theta) is an acute angle and sin (\theta) = (\frac{m}{n}), find cos (\theta)