1993 - JAMB Mathematics Past Questions and Answers - page 5
41
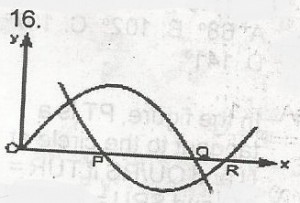
A
1, 3, 2
B
0, 0, 0
C
0, 2, 3
D
1, 2, 3
Ask EduPadi AI for a Detailed Answer
42
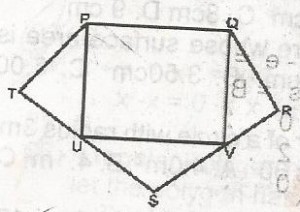
A
18o
B
54o
C
90o
D
108o
Ask EduPadi AI for a Detailed Answer
43
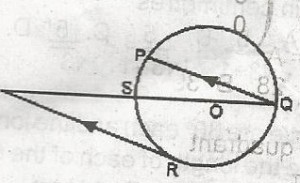
A
32o
B
45o
C
58o
D
90o
Ask EduPadi AI for a Detailed Answer
44
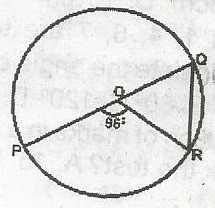
A
84o
B
48o
C
45o
D
42o
Ask EduPadi AI for a Detailed Answer
45
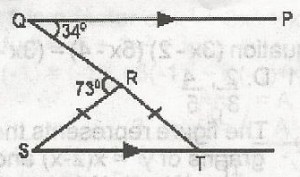
A
68o
B
102o
C
107o
D
141o
Ask EduPadi AI for a Detailed Answer
46
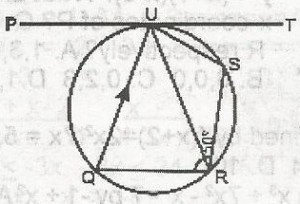
A
95o
B
85o
C
50o
D
35o
Ask EduPadi AI for a Detailed Answer
47
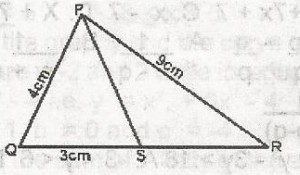
A
6\(\frac{3}{4}\)cm
B
3\(\frac{3}{8}\)cm
C
4\(\frac{3}{8}\)cm
D
2\(\frac{3}{8}\)cm
Ask EduPadi AI for a Detailed Answer
48
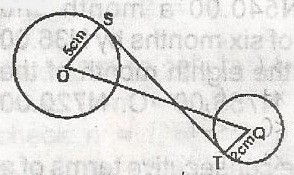
A
7 \(\sqrt{3}\)cm
B
12.9cm
C
\(\sqrt{87}\)cm
D
7cm
Ask EduPadi AI for a Detailed Answer
49

A
6cm
B
7cm
C
8cm
D
9cm
Ask EduPadi AI for a Detailed Answer
50

A
\(\frac{5}{\sqrt{3} + 1}\)
B
\(\frac{5}{\sqrt{3} - 1}\)
C
\(\frac{5}{\sqrt{3}}\)
D
\(\frac{\sqrt{3}}{5}\)
Ask EduPadi AI for a Detailed Answer