2001 - JAMB Mathematics Past Questions and Answers - page 6

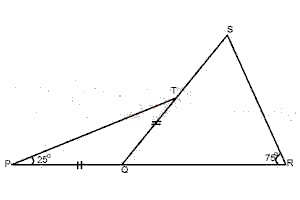
In Δ PQT,

∠PTQ = 25^o(base ∠s of isosceles Δ)

In Δ QSR,

∠RQS = ∠QPT + ∠QTP

(Extr = sum of interior opposite ∠s)

∠RQS = 25 + 25

= 50^o

Also in Δ QSR,

75 + ∠RQS + ∠QSR = 180^o

(sum of ∠s of Δ)

∴75 + 50 + ∠QSR = 180

125 + ∠QSR = 180

∠QSR = 180 - 125

∠QSR = 55^o

But ∠QSR and ∠RST are the same

∠RST = 55^o

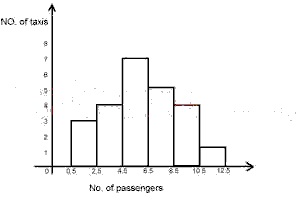
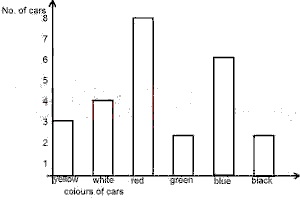
Number of yellow cars = 3

Total number of cars = 3 + 4 + 8 + 2 + 6 + 2 = 25

Fraction of yellow cars = ³/₂₅

< T = (\frac{x}{1}) = 25^o (PQ = QT)

< SQR = 2(25^o) = 50^o (sum of interior angle)

< Q + < R + < S = 180^o

50^o + 75^o + < S = 180^o = 125^o + < S = 180^o

< S = 180^o - 125^o = 55^o

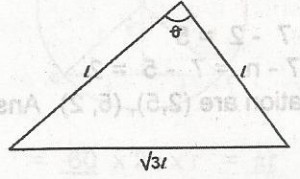
Using cosine formula (t(\sqrt{3}))² = t² + t² - 2t² cos(\theta)

3t² = 2t² - 2t² cos(\theta) = 2t²(1 - cos(\theta))

1 - cos(\theta) = (\frac{3t^2}{2t^2}) = (\frac{3}{2})

cos = 1 - (\frac{3}{2} = -\frac{1}{2})

(\theta) = cos^-1(-(\frac{1}{2})) = 120^o and 240^o

N.B 0 (\geq) (\theta) 360

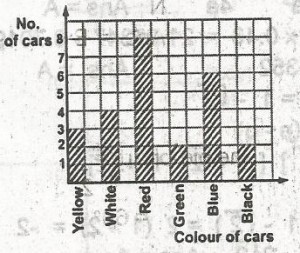
(\begin{array}{c|c} \text{colour of cars} & \text{Number (frequency)} \ \hline yellow & 3 \white & 4\ red & 8\ green & 2\ blue & 6\ black & 2\ \hline & 25 \ \hline\end{array})

Thus, the fraction of the total numbers that are yellow is (\frac{3}{25})

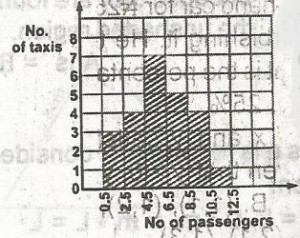
(\begin{array}{c|c} \text{no. of passengers} & \text{Number of taxis}\ \hline 0.5 - 2.5 & 3\ 2.5 - 4.5 & 4 \ 4.5 - 6.5 & 7\ 6.5 - 8.5 & 5\ 8.5 - 10.5 & 4 \ 10.5 - 12.5 & 1\ \hline \text{Total} & 24 \end{array})

Thus, the taxi with more than 4 passengers

= 7 + 5 + 4 + 1 = 17