2010 - JAMB Mathematics Past Questions and Answers - page 3

From the table, the number of students who failed the test is given as:

3 + 1 + 5 = 9

from the table, the number of students who took the test is 2 + 2 + 8 + 4 + 4 = 20

\(\begin{array} & Marks(x) & freq.(f) & fx \1 & 2 & 2 \ 2 & 2 & 4 \ 3 & 8 & 24 \ 4 & 4 & 16\ 5 & 4 & 20 \ \hline & \sum f = 20 & \sum fx = 66\end{array}\)
___________________________________

Mean mark ,\(\bar{x}\) = \(\frac{\sum fx}{\sum f}\)

= \(\frac{66}{20}\)

\(\bar{x}\) = 3.3

A committee of 2 women and 3 men can be chosen from 6 men and 5 women, in \(^{5}C_{2}\) x \(^{6}C_{3}\) ways

= \(\frac{5!}{(5 - 2)!2!} \times {\frac{6!}{(6 - 3)!3!}}\)

= \(\frac{5!}{3!2!} \times {\frac{6!}{3 \times 3!}}\)

= \(\frac{5 \times 4 \times 3!}{3! \times 2!} \times {\frac{6 \times 5 \times 4 \times 3!}{3! \times 3!}}\)

= \(\frac{5 \times 4}{1 \times 2} \times {\frac{6 \times 5 \times 4}{1 \times 2 \times 3}}\)

= 10 x \(\frac{6 \times 20}{6}\)

= 200

P(H) = \(\frac{1}{2}\) and P(T) = \(\frac{1}{2}\)

Using the binomial prob. distribution,

(H + T)³ = H³ + 3H²T¹ + 3HT² + T³

Hence the probability that three heads show in a toss of the three coins is H³

= (\(\frac{1}{2}\))³

= \(\frac{1}{8}\)

\(\begin{array}& x & x - \bar{x} & (x - \bar{x})^2 \2 & -2 & 4 \ 3 & -1 & 1 \ 5 & 1 & 1 \ 6 & 2 & 4\ \hline \sum x = 16 & & \sum (x - \bar{x}^2) = 0 \end{array}\)
___________________________________

\(\bar{x}\) = \(\frac{\sum x }{N}\)

= \(\frac{16}{4}\)

= 4

S = \(\sqrt{\frac {(x - \bar{x})^2}{N}}\)

= \(\sqrt{\frac {(10)}{4}}\)

= \(\sqrt{\frac {(5)}{2}}\)

Total cost = N34,000 + N2,000

= N36,000

15% = N115

\(\frac{115}{100}\) x N36,000

= N41,400

W \(\alpha\) U

W = ku

u = \(\frac{w}{k}\); \(\frac{2}{7}\) x \(\frac{3}{5}\)

= \(\frac{6}{35}\)

x(x - 1) > 0

x < -1 or x > 1

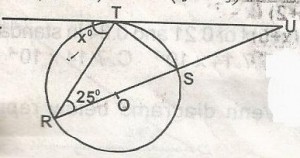
In the diagram above, < STU = < TRS = 25^o

(< between tangent to circle and a circle and a chord through the point of contact = < in the alternate segment)

< RTS = 90^o

(< in a semicircle = 90^o)
x^o + < RTS + < STU = 180^o

x^o + 90^o + 25^o = 180^o

x^o + 115^o = 180^o

x^o = 180^o - 115^o = 65^o