2014 - JAMB Mathematics Past Questions and Answers - page 5

Let E demote the event of obtaining at least a 4

Then n(E) = 16 + 10 + 14 = 40

Hence, prob (E) = (\frac{n(E)}{n(S)})

( = \frac{40}{100})

( = \frac{2}{5})

Sample space S = {10, 11, 12, ... 30}

Let E denote the event of choosing a number divisible by 3

Then E = {12, 15, 18, 21, 24, 27, 30} and n(E) = 7

Prob (E) = (\frac{n(E)}{n(E)})

Prob (E) = (\frac{7}{21})

Prob (E) = (\frac{1}{3})

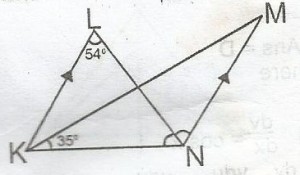
In the diagram above, (\alpha) = 54^o(alternate angles; KL||MN) < KNM = 2(\alpha) (LN is bisector of < KNM) = 108^o

35^o + < KMN + 108^o = 180^o(sum of angles of (\bigtriangleup))

< KMN + 143^o = 180^o

< KMN = 180^o - 143^o

= 37^o

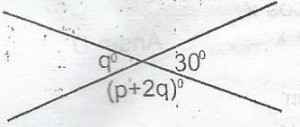
In the figure above, q^o = 30^o (vertically opposite angles)

(P + 2q)^o + 30^o = 180^o(angles on a straight line)

p + 2 x 30^o + 30^o = 180^o

p + 60^o + 30^o = 180^o

p + 90^o = 180^o

p = 180^o - 90^o

= 90^o

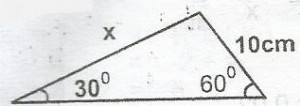
In the figure above, (\frac{x}{\sin 60^o} = \frac{10}{\sin 30^o}) (Sine rule)

x = (\frac{10 \sin 60^o}{\sin 30^o})

= 10 x (\frac{\sqrt{3}}{2} \times \frac{1}{2})

= 10 x (\frac{\sqrt{3}}{2} \times \frac{2}{1})

= 10(\sqrt{3})cm

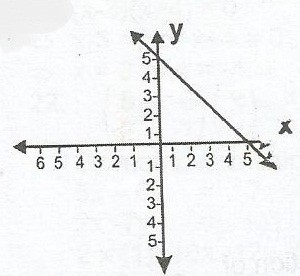
(x₁, y₁) = (0,5)

(x₂, y₂) = (5, 0)

Using (\frac{y - y_1}{y_1 - y_1} = \frac{x - x_1}{x_1 - x_1})

(\frac{y - 5}{0 - 5} = \frac{x - 0}{5 - 0})

(\frac{y - 5}{-5} = \frac{x}{5})

5(y - 5) = -5x

y - 5 = -x

x + y = 5

y = -x + 5

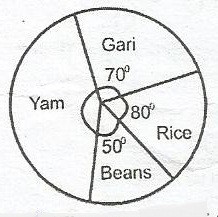
Angle of sector subtended by yam

= 360^o - (70 + 80 + 50)^o

= 360^o - 200^o

= 160^o

But (\frac{80^o}{360^o}) x T = 8000

T = (\frac{8000 \times 360^o}{80^o})

= N36,000

Hence the amount spent on yam = (\frac{160^o}{260} \times N36,000)

= N16,000